Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Nelson, Ray D.
and
Pope, Rulon D.
1998.
Improvements in the power of empirical stochastic dominance comparisons through kernel density estimation: a monte carlo study.
Journal of Statistical Computation and Simulation,
Vol. 60,
Issue. 4,
p.
295.
Maasoumi, Esfandiar
1999.
Handbook of Income Inequality Measurement.
p.
437.
Davidson, Russell
and
Duclos, Jean-Yves
2000.
Statistical Inference for Stochastic Dominance and for the Measurement of Poverty and Inequality.
Econometrica,
Vol. 68,
Issue. 6,
p.
1435.
Maasoumi, Esfandiar
and
Heshmati, Almas
2000.
Stochastic dominance amongst swedish income distributions.
Econometric Reviews,
Vol. 19,
Issue. 3,
p.
287.
Maasoumi, Esfandiar
2003.
A Companion to Theoretical Econometrics.
p.
538.
Barrett, Garry F.
and
Donald, Stephen G.
2003.
Consistent Tests for Stochastic Dominance.
Econometrica,
Vol. 71,
Issue. 1,
p.
71.
Roosen, Jutta
and
Hennessy, David A.
2003.
Tests for the Role of Risk Aversion on Input Use.
American Journal of Agricultural Economics,
Vol. 85,
Issue. 1,
p.
30.
Cebrián, Ana C.
Denuit, Michel
and
Scaillet, Olivier
2004.
Testing for Concordance Ordering.
ASTIN Bulletin,
Vol. 34,
Issue. 1,
p.
151.
Tse, Y. K.
and
Zhang, Xibin
2004.
A Monte Carlo investigation of some tests for stochastic dominance.
Journal of Statistical Computation and Simulation,
Vol. 74,
Issue. 5,
p.
361.
Lubrano, Michel
and
Protopopescu, Camelia
2004.
Density inference for ranking European research systems in the field of economics.
Journal of Econometrics,
Vol. 123,
Issue. 2,
p.
345.
Cebrián, Ana C.
Denuit, Michel
and
Scaillet, Olivier
2004.
Testing for Concordance Ordering.
ASTIN Bulletin,
Vol. 34,
Issue. 1,
p.
151.
Mugdadi, A.R.
and
Ahmad, I.A.
2005.
Moment inequalities derived from comparing life with its equilibrium form.
Journal of Statistical Planning and Inference,
Vol. 134,
Issue. 2,
p.
303.
Elbasha, Elamin H.
2005.
Risk aversion and uncertainty in cost‐effectiveness analysis: the expected‐utility, moment‐generating function approach.
Health Economics,
Vol. 14,
Issue. 5,
p.
457.
Linton, Oliver
Maasoumi, Esfandiar
and
Whang, Yoon-Jae
2005.
Consistent Testing for Stochastic Dominance under General Sampling Schemes.
Review of Economic Studies,
Vol. 72,
Issue. 3,
p.
735.
Maasoumi, Esfandiar
Millimet, Daniel L.
and
Rangaprasad, Vasudha
2005.
Class Size and Educational Policy: Who Benefits from Smaller Classes?.
Econometric Reviews,
Vol. 24,
Issue. 4,
p.
333.
Maasoumi, Esfandiar
and
Millimet, Daniel L.
2005.
Robust inference concerning recent trends in US environmental quality.
Journal of Applied Econometrics,
Vol. 20,
Issue. 1,
p.
55.
2006.
Poverty and Equity.
Vol. 2,
Issue. ,
p.
289.
Carrington, Anca
2006.
Regional Convergence in the European Union: A Stochastic Dominance Approach.
International Regional Science Review,
Vol. 29,
Issue. 1,
p.
64.
Ahmad, I.A.
Ahmed, A.
Elbatal, I.
and
Kayid, M.
2006.
An aging notion derived from the increasing convex ordering: the NBUCA class.
Journal of Statistical Planning and Inference,
Vol. 136,
Issue. 3,
p.
555.
Xu, Kuan
and
Fisher, Gordon
2006.
Myopic loss aversion and margin of safety: the risk of value investing.
Quantitative Finance,
Vol. 6,
Issue. 6,
p.
481.
 , for all x. Second-order stochastic dominance plays an important role in economics, finance, and accounting. Here a statistical test has been constructed to test
, for all x. Second-order stochastic dominance plays an important role in economics, finance, and accounting. Here a statistical test has been constructed to test 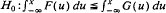 , for some x ∈ [a, b], against the hypothesis
, for some x ∈ [a, b], against the hypothesis  , for all x ∈ [a, b], where a and b are any two real numbers. The test has been shown to be consistent and has an upper bound α on the asymptotic size. The test is expected to have usefulness for comparison of random prospects for risk averters.
, for all x ∈ [a, b], where a and b are any two real numbers. The test has been shown to be consistent and has an upper bound α on the asymptotic size. The test is expected to have usefulness for comparison of random prospects for risk averters.



